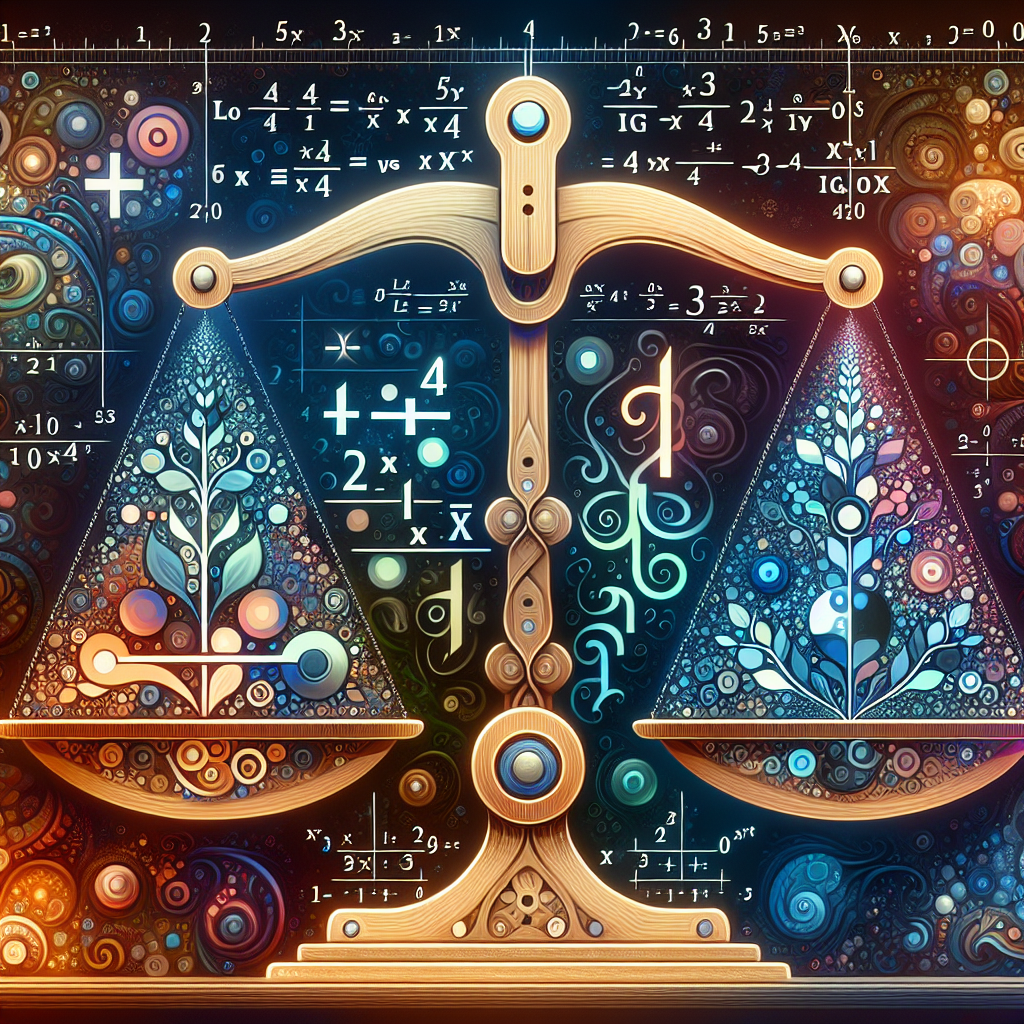
log4(2x+4)-3 =log4 3
log4(2x+4) - 3 =log4 3
log4(2x+4) - 3log44 = log4 3
log4(2x+4) - log4 4^3 = log43
log4[(2x+4)/64] = log43
(2x+4)/64 = 3
2x+4 = 192
2x=188
x = 94
Well, it looks like you have a math equation there. I suppose you could call it logarithmetic! But hey, I'm here to clown around and not solve equations. So let me tell you a joke instead:
Why don't scientists trust atoms?
Because they make up everything!
Hope that brought a smile to your face! If you still need help with your equation, I'd recommend asking a math expert.
To solve the equation log4(2x+4) - 3 = log4 3 step-by-step, follow these steps:
Step 1: Combine the two logarithms on the left side of the equation using the logarithmic identity: loga(x) - loga(y) = loga(x/y).
log4(2x+4) - 3 = log4 3
log4((2x+4)/4) = log4 3
Step 2: Simplify the logarithmic expression by canceling out the common base.
(2x + 4)/4 = 3
Step 3: Multiply both sides of the equation by 4 to eliminate the fraction.
2x + 4 = 12
Step 4: Subtract 4 from both sides of the equation to isolate the variable.
2x = 8
Step 5: Divide both sides of the equation by 2 to solve for x.
x = 4
Therefore, the solution to the equation log4(2x+4) - 3 = log4 3 is x = 4.
To solve the equation log₄(2x+4) - 3 = log₄ 3, we need to isolate the variable, x.
Step 1: Combine the logs using the properties of logarithms
Using the property logₐ(b) - logₐ(c) = logₐ(b/c), we can combine the two logarithms on the left side of the equation.
log₄(2x+4) - 3 = log₄ 3
log₄(2x+4)/3 = log₄ 3
Step 2: Remove the common logarithm base
Since the base of the logarithm on both sides of the equation is the same (4), we can remove the logarithm and set the arguments equal to each other.
(2x+4)/3 = 3
Step 3: Solve for x
We can now solve the equation:
2x + 4 = 3 * 3
2x + 4 = 9
2x = 9 - 4
2x = 5
x = 5/2
Thus, the solution to the equation is x = 5/2.